Vertex Dividing-Head – basic help please

[Nigel Graham 2Participant @nigelgraham2]

I have acquired a Warco-badged Vertex BS-0 Dividing-head in immaculate condition, probably unused by its previous owner, a fellow club-member, before he passed away.

It came with tailstock but no chuck (not a problem as Myford chucks fit), T-slot tenons or instructions.

What I think is meant to be the official manual, on-line, is a pdf image of hopeless image quality, impossible to down-load, with illegible parts-diagrams and lacking key operating details. The text mentions parts that seem not to exist, at least not on this model.

So, now I want to use the thing…. Help please, on its basic use.

I have found so far…

Changing the division plates – I worked that out by careful experimenting. I probably have generic instructions on using dividing-heads, but the pdf is short on identifying this one's individual controls.

Of two levers on the side, one controls the the indexing-pin for direct indexing; the other, unidentifiable on the document, may operate the spindle lock.

The text gives vague information on taking up backlash in the worm, from wear, but the photos are uselessly fuzzy.

For direct indexing it instructs ' swing the worm out of engagement ', but not how! Removing the division-plate reveals a bronze disc with two cap-screws working through curved slots. The purpose is not clear and the screws are so tight I stopped, fearing damaging the tools or worse, the unit.

For " Indirect indexing " (I.e. actual dividing) it tells you to ' swivel the worm carefully to the stop by means of the swivel lever, at the same time turning slightly the indexing spindle then the hand crank… Operate clamping device for hole plate.'

Which ' stop ', ' swivel lever ' and ' clamping device for [which] hole plate ' ? Note that fitting the dividing-plate covers the slotted bronze disc and its screws. The rest of the instructions for this section are just as baffling, and the diagrams are barely readable.

So I will be very grateful if someone can elucidate, please!

[Nigel Graham 2Participant @nigelgraham2]

[Neil LickfoldParticipant @neillickfold44316]

Tis place has a download of instructions.

https://www.machineryhouse.co.nz/D001?gclid=CjwKCAiArbv_BRA8EiwAYGs23LHabYJ-rwlM0CG5svqBKIm3L2fbYo9LEiKssQx8LmOzP1F0_iSFiBoCy0UQAvD_BwE

Is that the same unit?

There is a lever on the side that engages or disengages the worm. The other lever normally is the index pin engagement or disengages the quick select on the index plates, or is the spindle lock.

[HopperParticipant @hopper]

Or Here:

CHRONOS

[John OlsenParticipant @johnolsen79199]

The other lever on the side is as you thought the spindle lock.

The bronze piece with the curved slots is how you would adjust the backlash and take the worm out of engagement. For direct indexing I find it easier to leave the worm in engagement, and just wind the handle until the pin will engage in the hole.

I think the part about indirect indexing is just telling you to get the worm engaged with the worm wheel, you would do this before putting on the dividing plate. The main thing is to have the worm engaged well enough to not have too much backlash, but not so tight that it starts to bind. The two screws in the curved slots should be allan screws, and should not need to be done up excessively tight.

They tend not to come with T slot tenons since they have to fit the particular milling table, which may not have T slots the same width.

I have the original Vertex instructions for mine. I could dig them out and scan them. Mine is of about eighties vintage and does not take Myford chucks, more's the pity, although screw on chucks are not ideal for milling. I was lucky enough to acquire a spare three jaw which now fits it, and I also have a faceplate for it. The taper is a Brown and Sharp, also a bit obscure. With any luck, yours might be a Morse taper.

John

[DC31kParticipant @dc31k]

Would you be able to copy and paste the following into Google?

bs0 dividing head manual

There are many sets of instructions, the US-Grizzly ones are normally good. If reading is not your thing, there are some good videos on YouTube.

It would be prudent to do an independent check on the numbers given in the division tables. There have been posts here that the ones supplied with the rotary table dividing plates are incorrect.

[Nigel Graham 2Participant @nigelgraham2]

Thank you!

Neil –

Yes, that's the one.

The upper lever does, as you say, operate the direct indexing pin. I don't know if it also locks the spindle.

The handle lower down and further back, doesn't do anything on mine – except unscrew from whatever it engages if I wind it back far enough. It is feasible the unit has never been used, and that part of its innards is stuck on gummed-up oil, although the spindle turns smoothly enough from the worm-drive handle.

'

John –

Ooh, yes, thank-you for the offer. That would be very helpful! I don't know the source of what I copied, but it looks as if from the (Taiwanese?) manufacturers. The standard of English is high though not quite right in places, but the contents don't identify the details sufficiently to make sense.

They do imply that the disc behind the dividing-plate is only for adjustment. Its Allen screws are probably tight by original assembly plus external oil or preservative "varnish".

The worm is engaged, with the disc apparently at full angular travel, as I can turn the work spindle from the worm spindle without problems.

 

Most of the work it will do for me is making regular polygons where I want high accuracy, not the gear-cutting I would like to try. So though using the worm-drive is just as I do with my fixed-worm rotary table*, it makes sense to use direct indexing to minimise wear on the worm drive.

The taper is hard to identify and looks very short, but as the unit is made to take Myford (and presumably Warco!) chucks it probably is Morse. I can test it with a taper tool and marking-blue.

I know from using rotary tables the problem with screwed-on chucks and milling.

I have measured the tenon grooves, still gummed up with preservative, and the milling-machine T-slots, and have some suitable material. I'd also need make a pair for the horizontal mill – but that machine itself still needs fully installing!

'

*The rotary table enabled me to rough out for turning, a two-throw crankshaft made from solid; but is not designed to be used edge-on, necessitating clumsy great angle-box mountings.

'

Hopper –

I'll try that. The unit is actually badged Warco!

'

Dc31K –

I'll have a look. That is almost exactly what I used, so I  might end up with the same thing.

 

Edited By Nigel Graham 2 on 02/01/2021 10:44:31

[Simon Williams 3Participant @simonwilliams3]

Slightly late to the party – but if it helps –

I've got the same dividing head, and can confirm that there are indeed two levers at the back face. The top one operates a spindle locking pin which engages with the circle of holes in the face plate, exactly as described above.

The second lower lever screws in until it is tight, by which time it has locked the spindle solid.

To the best of my knowledge (though you've got me wondering now) there is no mechanism for taking the worm out of mesh with the pinion.

HTH Simon

[Simon Williams 3Participant @simonwilliams3]

Well, I did think of editing my previous post so I could deny I ever made a fool of myself, but no-one would be convinced.

So my apologies for misinformation, but having just had a play in my (cold and damp) shed I can confirm that there is indeed a slotted plate behind the dividing plate, which, after loosening two cap head screws, permits the worm to be disengaged from the pinion.

It's a bit of a fiddle to set it back where it belongs with minimum backlash, but it does exist.

Shows how much I know!

 

edited for typo.  This isn't going well…

Edited By Simon Williams 3 on 02/01/2021 11:56:27

[Nigel Graham 2Participant @nigelgraham2]

Thank you Simon.

The instructions, such as they are, imply a lever for disengaging the worm. It's possible they are written generically for the whole range. The larger Vertex units are designed for driving the spindle from the milling-machine so may have a defined "gear lever".

I'll treat mine to a bit of cleaning with white spirit to remove the solidified traces of preservative from around the screw-heads, and try that.

At least it's not in my cold and hopefully dry shed, but on a rack here with me in the slightly warmer and drier front room.

DC31K –

That link, and the Chronos one that Hopper suggests, raise the document I'd already found.

[Nigel Graham 2Participant @nigelgraham2]

Progress!

I cleaned and lubricated the slotted disc that had been puzzling me, and found that indeed it does disengage the worm from its wheel.

The main spindle is a bit stiff and "lumpy" to turn, which is probably a result of it standing idle for a long time.

So that's the first question sorted. I can now use the head for direct indexing. What of full dividing?

'

The on-line manual's section on dividing is as clear as oily swarf, by poor technical-authorship plus the image seeming to be a low-quality colour photocopy of a printed page, rather than a DTP-file saved directly as pdf.

However…

I found M-Machine has some useful information-sheets on its web-site, and one covers basic dividing-head calculations. It is written and illustrated clearly but I am not right good with numbers so I'll have to read it very carefully and try their worked examples; but I think we'll get there. I may have explanations in text-books, too.

Thank you all, chaps!

[HopperParticipant @hopper]

Posted by Nigel Graham 2 on 02/01/2021 23:43:49:…

…'

The on-line manual's section on dividing is as clear as oily swarf,

You need Harold Hall's book "Dividing". Its a Workshop Practice Series book, so as inexpensive as it is informative. It will give you a run through on the basics of using a dividing head, pitched at beginner level and going to some quite advanced stuff.

First thing you need to determine is the reduction ratio of your dividing head. Either count the number of teeth on the worm wheel inside it, or count the number of turns of the handle required to rotate the spindle by one turn.

Once you have determined if you have a 60 or 90 or 40 to 1 ratio dividing head, you can then look up the dividing chart for that ratio online or in various books, including the above mentioned.

These charts will tell you which circle of holes to use on the indexing plate and how many turns of the handle plus how many holes in that particular circle are required to achieve the number of divisions (or gear teeth etc) you want. The two moveable "fingers" on the index plates are used to quickly set the correct number of holes rather than counting holes at each and every adjustment.

No need to calculate from first principles, although it is good to know the theory behind the charts.

Edited By Hopper on 03/01/2021 00:39:03

[not done it yetParticipant @notdoneityet]

Once you have selected the suitable hole plate and the hole circle, it is dead easy.

However many turns required to give you a full 360 degrees rotation of the table means you can easily calculate the total number of holes (spaces) that pass by in one complete 360 degree turn.

That total number divided by the number of teeth gives you the number of holes (or spaces) per single tooth.

Convert that number of holes (spaces) into a whole number and the remainder (for the number of holes in the plate circle used) – only if the number exceeds the number of holes in the selected plate circle – and you have the number of turns (may be zero) and the number of holes (spaces) required for each tooth setting.

[Martin ConnellyParticipant @martinconnelly55370]

Harold Hall's website has a primer on this subject and also shows the details of his book.

Using a dividing head

Martin C

[Simon Williams 3Participant @simonwilliams3]

And when you set the fingers, be sure to span one more than the arithmetic formula (look up tables) tell you as the first hole (zero'th hole) doesn't count. That's the significance of NDIY's reference to counting spaces on the dividing plate. So if you need to move the worm 20/40 of a turn (assumes a 40 : 1 reduction) the fingers embrace 21 holes or 20 spaces.

Harold Hall's book explains it all.

[not done it yetParticipant @notdoneityet]

First hole is number zero. I’ve seen so many instances where students start their stopwatches counting at one, not zero for their experiments! You are simply not allowed to count that first hole twice, so zero is equivalent to 360 for the full turn.

Same as counting time. Midnight is 00:00:00 so the start of the day and that rolls round to 23:59:59 at the end of the day (there is no 24:00h in most time systems and certainly not in the SI system – some systems , like a ‘24 bells’ one in the military, would have to ring 24 bells because nobody would hear the ‘no bells’ signal!).

Edited By not done it yet on 03/01/2021 11:39:24

[John ATTLEEParticipant @johnattlee20632]

Dear All,

I have very recently bought a Vertex BS 1 from RDG tTools. I have found it to be a lovely machine and have already made a 25 and 55 teeth spur gear for my lather in order to screw cut. The literature was fine, albeit with typical errors, and even I could understand it! I think that Harold Halls book is essential.

I made up an arbor from a morse taper blank that are cheap enough. I did have to extend it by locktiting an extension shaft which was then machined to suit. I was careful to machine the teeth so that the cutting forces pushed the MT arbor into the dividing head. I supported the end of the arbor extension with the adjustable tails stock.

I don't think that a chuck is essential because it is easy to secure a mechanical drive connection to the backplate and machine between centres. I think that a MT setting bar would be useful. Instead, I clocked the side of the dividing head to set it up square.

I have an indexing head but if I was using the dividing head for 'indexing' I don't think I would try to disconnect the worm.

On an important job (like my 55 teeth gear wheel) I did do a dummy run marking the indexing plate with pencil to check that I had got it set up correctly.

In short, a great bit of kit!

John

[Howard LewisParticipant @howardlewis46836]

Having had problems with my Vertex HV6 Rotary Table (or the chart supplied with it ) I spent some time making up an EXCEL spreadsheet, to calculate the Turns and Holes for various divisions. A lot of the results are unuseable, requiring partial holes but others are useable.

It is easy to extend the spreadsheet, by inserting a line and typing in the required number of divisions.

For Tables / Dividing Heads with ratios other than 90;1, changing one of the formulae, and copying down through the spreadsheet should provide the required answers.

I think that Neil posted it under the "Model Engineers Workshop Articles" heading about the HV6, in about 2016.

It might be of help?

Howard

[Nigel Graham 2Participant @nigelgraham2]

Thank you for the advice on the calculations.

I think I will have to treat myself to Harold Hall's book, as Hopper suggests. I have his books on milling and on tool & cutter grinding so am confident of his ability to explain things.

I also have Ivan law's books on milling and on gear-cutting. The former apparently assumes most model-engineers making gears would need only numerically straightforward tooth-counts; but does show the versatility of rotary-tables and dividing-heads generally. The latter book however, does describe setting a dividing-head to give the wanted count, by a worked example.

If you needed only ever to make a few gears, especially ones with awkward numbers, you could adopt the trade practice by buying pilot-bored gears and modifying their centres to suit. It may even be cheaper than the costs of blanks and cutters. That would not suit all applications though, and of course if for a model more so than for a machine-tool accessory, you've evaded the challenge!

All those book are in the 'Workshop Practice' series, and I know I am not the only one to have recommended appropriate choices from it to other enquirers on this forum.

I looked in my copy of Stan Bray's Milling (a Crowood publication).. Rather surprisingly perhaps, whilst a very helpful text in other respects, he devotes almost nothing to dividing-head calculations. I think he reflects the more common and perfectly good practice of making simple or compound, direct indexing-heads that borrow change-wheels from the lathe.

Some of my "full-size engineering" texts may explain the sums, but are likely to be far harder to follow.

'

John Attlee –

Did your dividing-head come with a properly printed manual? The problems with those I found on-line one were not only poor explanations but also very poor reproduction, on screen as well as in printing.

[Michael GilliganParticipant @michaelgilligan61133]

Nigel,

You may find this useful: **LINK**

http://vintagemachinery.org/pubs/detail.aspx?id=5863

… it’s quite a decent quality scan.

MichaelG.

.

Edit: and a copy [better than some] of the Vertex manual is here:

https://www.t4i.com.au/D001

Edited By Michael Gilligan on 04/01/2021 00:06:03

[Simon Williams 3Participant @simonwilliams3]

Anything's better than Eastenders – so

Exploring the delights of Youtube I chanced upon these:

overview BS0 Dividing head

Taking one to bits

Enjoy!

HNY Simon

PS I think the black oiliness is probably molyslip type oil rather than wear, but I could be wrong. Again. Not that I've got any hang-ups.

[BERT ASHTONParticipant @bertashton57372]

I have a photostat copy of the B & S dividing head manual.

If anybody wants a copy inbox me.

[not done it yetParticipant @notdoneityet]

What is it with people and simple maths!

Take a somewhat simplified example.

Say 1). the table turns is 90 per full revolution

2). Ten teeth are required.

Very clearly the answer will be 10 turns per tooth, but lets do the calculation, just as a demonstration….

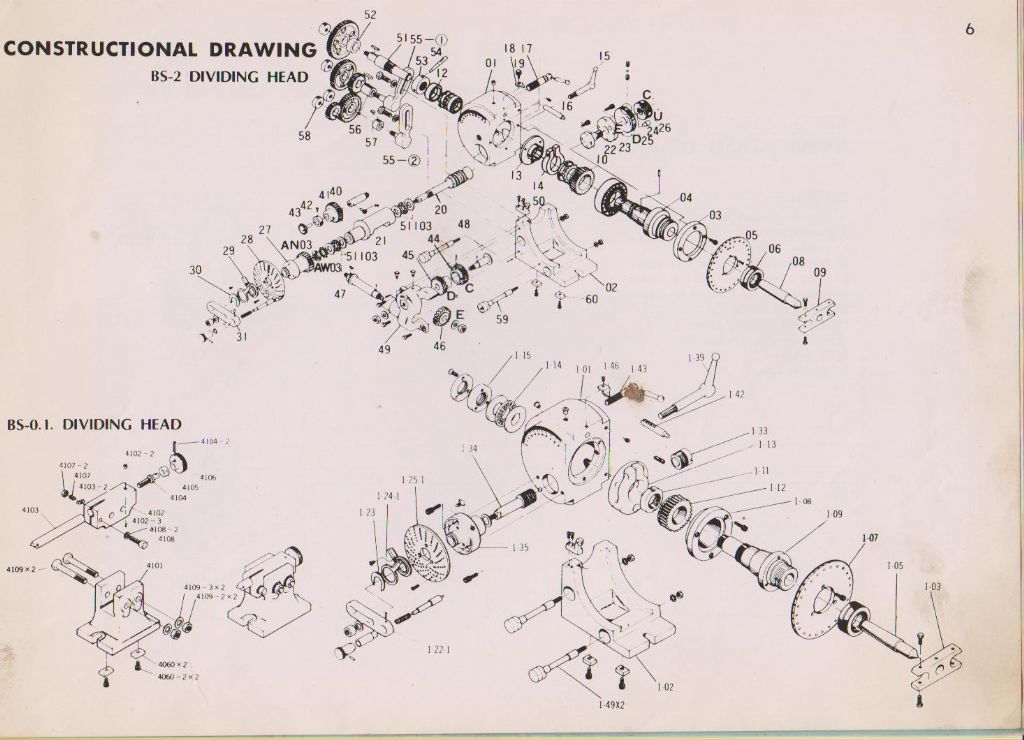
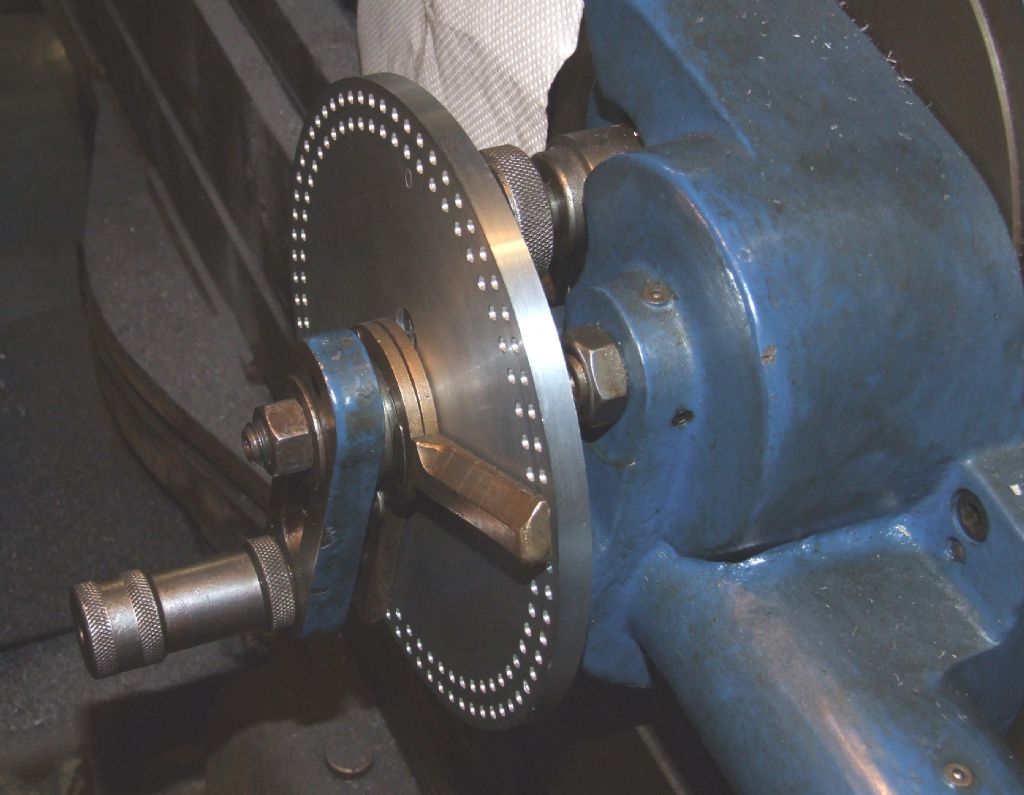
Clearly we would want to choose a 10 hole (space) plate for this.🙂

For 90 turns of the handle 900 holes would pass the starting point (simple enough for everyone? 90 x 10 = 900)

if 900 holes is turned for 10 teeth, one tooth would need 90 holes (that’s right, 900/10 = 90).

We know that each turn of the handle is 10 holes, so 90 holes is 90/10 turns. = 9 turns. Surprise, surprise?

ANY AND EVERY CALCULATION IS NO DIFFERENT. MOST HAVE A CALCULATOR TO ACTUALLY DO THE MATHS. SO WHY DO WE HAVE TO KEEP GOING OVER THIS SUBJECT? (the answer is below)

—————-

Some more examples, a little more challenging:

say 19 teeth with a 40 turn rotary table/dividing head (an unlikely real life example)

We clearly choose a 19 hole plate for this, so 40 turns is 40 x 19 holes in total (= 760)

760 holes has to make 19 teeth so each tooth will require 760/19 holes = 40 holes per tooth.

With a 19 hole plate that will be 2 turns and 2 holes for each tooth. JOB DONE. One could use a plate circle of 38 or 57 and both would work (but unlikely those numbers would be found on your dividing plates🙂 )

Now, if a 38 tooth plate was used the answer would work out to 2 complete turns and 4 holes. Exactly the same result in practice.

The simplest maths is: take table turns ratio, multiply by holes in plate, then divide by the number of teeth required. If the answer is greater than the hole number chosen it will need more than one turn and you can work out how many and the remainder.

Another: 45 teeth with a 72 turn table

Let’s choose a 15 hole circle (as that is a factor of 45 (15 x 3 = 45)). There unlikely to be a 3, 5, 9 or 45 hole circle on your plates.🙂

72 turns of 15 holes = 72 x 15 = 1080 holes for 45 teeth.

1080 divided by 45 will give holes per tooth = 24

(The calculator operations needed are 72 x 15/45 = 24)

We have a 15 hole circle so require one complete turn and 9 holes per tooth. Job done.

Notes: if your correctly calculated answer does not equal an exact whole number, you’ve chosen the wrong plate circle! Prime numbers are always included on the plate circles or they can not be cut.

———-

Only three numbers to be inputted to one’s calculator, basically. Nothing is difficult about that. BUT choosing a suitable plate circle seems likely to be the major stumbling block? (The answer to the question posed above).

Lets look at a 63 tooth gear. Possible factor pairs of 63 are (1, 63) (3, 21) and (7, 9).

A 63 hole plate would be easy (one hole/tooth), but 63 hole plates are thin on the ground. 1 and 3 are not sensible choices so 7, 9 and 21 are possibilities (even though 7 and 9 are not usually found on plates). Just trying them with your table turns, to see if the answer is an exact whole number, is one way to find a possible solution.

90 turn plate:

90 x 7/63 = 10 Works OK but unlikely an option

90 x 9/63 = 12.86 Does not work out to a whole number, so not OK.

90 x 21/63 = 30 Works OK

72 turn plate:

72 x 7/63 = 8 Works OK but not likely an option

72 x 9/63 = 10.29 Does not work out to a whole number, so not OK

72 X 21/63 = 24. Works OK

40 turn table:

40 x7/63 = 4.44 Does not work out to a whole number, so not OK

40 x 9/63 = 5.71 Does not work out to a whole number, so not OK

40 x21/63 = 13.3 Does not work out to a whole number, so not OK

(with a 40 turn table one would require a 63 hole plate!).

36 turn table:

36 x7/63 = 4 Works OK but not likely an option

36 x 9/63 = 5.14 Does not work out to a whole number, so not OK

36 x 21/63 = 12 Works OK

Note that for all the OKs, the ‘other’ factor is also a factor of the table turns ratio.🙂 Helps when deciding on a plate circle to check out. As one’s table will be of a known turns ratio, you would soon work out the possible options fairly easily. The plates are made with the least number of useful circles (why 7 and 9 are not usually found) and will include all possible prime numbers. Numbered hole circles will work for any turns ratio for that same number of teeth – the answer for the number of holes/tooth will be the same as the turns ratio (no calculation needed).

I included the 40 turns ratio table examples to demonstrate why they are not a popular choice (9 degrees per turn of the handle!) for either the user or (more importantly) the manufacturers🙂 .

This boils down to: pick the highest factor of the tooth count, that is on your plates, and just work it out.

Remember that holes really means spaces in all the above…

I didn’t get a chart with either of my rotary tables and I would not trust them (or the internet) when it is so easy to sort it out myself. I use so few settings that keeping a chart for it would be pointless for me.

[Anonymous]

Posted by not done it yet on 04/01/2021 09:57:40:

………..but 63 hole plates are thin on the ground.

For my traction engines I needed to cut 63 and 69 tooth gears, neither value is listed under simple indexing for my industrial 40:1 dividing head. My dividing head is universal, but rather than set up compound or differential indexing it was easier to make a 63 and 69 hole plate:

Andrew

[Nigel McBurney 1Participant @nigelmcburney1]

I dont see how making a two row index plate is easier than setting the differential indexing gear train I assume cnc was used to space out the holes in a new plate,but there is still the steel to locate,turn to size and drill and c/bore the screw holes. I last used differential indexing for a customer job a couple of years ago that was a 63 t gear .

[Author]

Posts