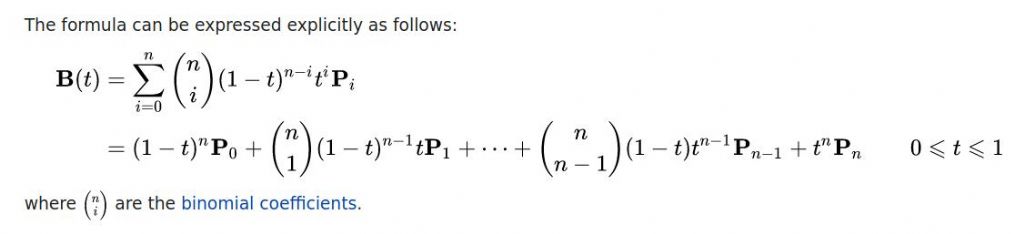Old School Drawing Exercises and 2D CAD
Old School Drawing Exercises and 2D CAD
- This topic has 145 replies, 22 voices, and was last updated 10 August 2022 at 19:07 by
Alyn Foundry.
- Please log in to reply to this topic. Registering is free and easy using the links on the menu at the top of this page.
Latest Replies
Viewing 25 topics - 1 through 25 (of 25 total)
-
- Topic
- Voices
- Last Post
Viewing 25 topics - 1 through 25 (of 25 total)
Latest Issue
Newsletter Sign-up
Latest Replies
- Dodgy car parts a new low
- ELECTRICITY IN SPAIN & PORTUGAL
- Lathe floor height?
- Selling an Expensive Item
- Identifier
- moving 150kg+ machines inside warehouse
- 24cc DIESEL ENGINE FROM SOLID
- Some help with a Cowells speed controller needed.
- Anyone know about wells and Victorian plumbing?
- Power hacksaw – powerful banging when running