Isochronous knife edge suspension?

Advert

New Replies

Report Bug

Help/FAQs

Isochronous knife edge suspension?

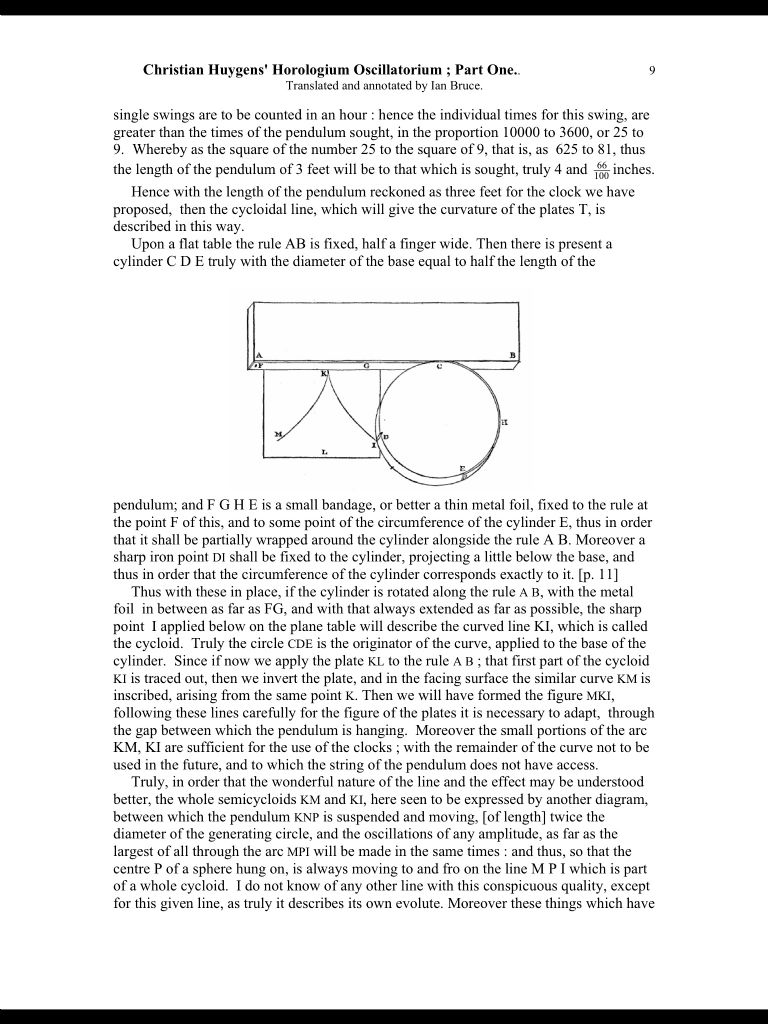
This topic has 141 replies, 8 voices, and was last updated 15 September 2023 at 16:38 by John Haine.

Viewing 25 posts - 1 through 25 (of 142 total)

1 2 3 4 5 6 →

Author

Posts
26 August 2023 at 21:59 #658112
david bennett 8
Participant
@davidbennett8
I was idly considering knife edges when it occured to me that a cylinder on two parallel knives would represent a circle rolling on a plane – almost the perfect description of a cycloid. I imagine this has been looked into before. Any suggestions where to look? The pic is just the general idea, but with the knives under the "pivots" to reduce rolling.(please view with bob at the bottom)

dave8

Edited By david bennett 8 on 26/08/2023 22:01:21

Edited By SillyOldDuffer on 27/08/2023 10:45:27
Advert

26 August 2023 at 21:59 #4034
david bennett 8
Participant
@davidbennett8
26 August 2023 at 22:12 #658114
Michael Gilligan
Participant
@michaelgilligan61133
A nice friendly Moderator might rotate that picture for you, Dave

Meanwhile … I think the first problem is that the radius of your cylinder is too small

For the geometry to work, that radius would be the same as the length of the pendulum

MichaelG.
26 August 2023 at 22:27 #658118
david bennett 8
Participant
@davidbennett8
Posted by Michael Gilligan on 26/08/2023 22:12:53:

A nice friendly Moderator might rotate that picture for you, Dave

Meanwhile … I think the first problem is that the radius of your cylinder is too small

For the geometry to work, that radius would be the same as the length of the pendulum

MichaelG.

Doh! i'm sure you are right, but wii have to work on getting my head round it.

dave8
26 August 2023 at 22:52 #658122
Michael Gilligan
Participant
@michaelgilligan61133
I oversimplified, Dave … but this should give you a good sense of the required geometry

**LINK**

https://iopscience.iop.org/article/10.1088/1361-6552/acd612/pdf

It’s recent, and I’ve only just found it, so will be reading at breakfast.

MichaelG.
26 August 2023 at 22:56 #658123
John Haine
Participant
@johnhaine32865
This has been analysed by Philip Woodward but alas doesn't result in isochronism. I could dig out the reference if interested.
27 August 2023 at 00:16 #658130
david bennett 8
Participant
@davidbennett8
Posted by John Haine on 26/08/2023 22:56:53:

This has been analysed by Philip Woodward but alas doesn't result in isochronism. I could dig out the reference if interested.

Thanks for the offer John, but I can now see that the correction should be a proportion of the pendulum length. In my idea, the correction would be in proportion to the roller radius, thus incorrect.

dave8
27 August 2023 at 07:37 #658134
John Haine
Participant
@johnhaine32865
Well for completeness it was in HJ August and September 1994.

Intriguingly, he shows that if the roller rolled below a flat rolling surface it could work. Very strong magnets might do the trick, but then the rest of the pendulum would have to be non-magnetic to avoid magnetic forces interfering with gravitational.

Edited By John Haine on 27/08/2023 07:41:55
27 August 2023 at 10:56 #658164
SillyOldDuffer
Moderator
@sillyoldduffer
I suspect implementation problems would make this difficult to build.
- If the edges weren't perfectly horizontal, the roller would tend to walk downhill
- If the edges were perfectly horizontal but not perfectly parallel, the roller would tend to walk towards the narrow end
- If the edges weren't perfectly horizontal and parallel, the pendulum would swing in an ellipse
- As above unless the roller is a perfect cylinder – circular, with no taper
Got to be worth trying though. Perfect is the enemy of good!

Dave
27 August 2023 at 12:35 #658183
gerry madden
Participant
@gerrymadden53711
Posted by John Haine on 27/08/2023 07:37:48:

Well for completeness it was in HJ August and September 1994.

…….. but then the rest of the pendulum would have to be non-magnetic to avoid magnetic forces interfering with gravitational.

He could still get 'inductive damping' (as in magnetic compasses), due to eddy currents
27 August 2023 at 14:57 #658211
david bennett 8
Participant
@davidbennett8
Interestingly, when this idea came up I was playing about with these magnets and (steel) coins., and a steel plate.

dave8
28 August 2023 at 10:43 #658281
david bennett 8
Participant
@davidbennett8
Posted by Michael Gilligan on 26/08/2023 22:12:53:

A nice friendly Moderator might rotate that picture for you, Dave

Meanwhile … I think the first problem is that the radius of your cylinder is too small

For the geometry to work, that radius would be the same as the length of the pendulum

MichaelG.

Michael, are you sure? I cannot reconcile this. Surely a roller of any size on a flat plane must produce a cycloid. The bob must follow this shape.

dave8
28 August 2023 at 11:29 #658289
John Haine
Participant
@johnhaine32865
The actual locus of the bob is a "prolate trochoid", not a cycloid. Woodward shows that the radius of curvature of this for small angles is greater than the circular path of the bob with no roller. This means that the "lift" of the bob for a given deflection is less than for a circle, whereas for a true cycloidal path it would be greater. In fact the circular deviation of a pendulum with roller suspension is greater rather than less than normal. Others have confirmed this numerically. Unless one could hang the roller on a magnet this means that there's no point in roller suspensions in an attempt to reduce circular deviation.

Another point is that for compound pendulums (which all real pendulums are to a greater or lesser extent), it can be shown that there is no path the bob could follow that will be isochronous. There are some schemes to correct for circular deviation over a small range of angles, of which the most successful was probably the Fedchenko suspension spring. Or one can try to control CD to gain other benefits which is what Harrison's circular cheeks do.
28 August 2023 at 12:33 #658296
david bennett 8
Participant
@davidbennett8
John, yes I can see that. I was viewing it as a "negative cycloid" But I was questioning Michaels assertion that the roller size mattered. I am experimenting with magnets.

dave8

Edited By david bennett 8 on 28/08/2023 12:38:57
28 August 2023 at 15:50 #658313
Michael Gilligan
Participant
@michaelgilligan61133
Posted by david bennett 8 on 28/08/2023 10:43:02:

Posted by Michael Gilligan on 26/08/2023 22:12:53:

A nice friendly Moderator might rotate that picture for you, Dave

Meanwhile … I think the first problem is that the radius of your cylinder is too small

For the geometry to work, that radius would be the same as the length of the pendulum

MichaelG.

Michael, are you sure? I cannot reconcile this. Surely a roller of any size on a flat plane must produce a cycloid. The bob must follow this shape.

dave8

.

To be honest, Dave … no I’m not sure:

… It was a knee-jerk reaction, and I would be perfectly happy to be proved wrong.

… I was just thinking back to Huygens’ original, which is lodged firmly in my mind.

Please experiment, and please advise !!

MichaelG.

.

This is not it … but is a delightful image:

https://commons.wikimedia.org/wiki/File:Cycloidal_pendulum_demonstration.png

Edited By Michael Gilligan on 28/08/2023 15:55:56
28 August 2023 at 16:15 #658315
Michael Gilligan
Participant
@michaelgilligan61133
.

Ian Bruce’s translation ^^^ is well-worth reading

If you can’t find it on t’internet … send me a PM with your eMail address and I will send you the PDFs

MichaelG.
28 August 2023 at 18:28 #658323
david bennett 8
Participant
@davidbennett8
(general post)

Hopefully, this will help with visuslising the problem with mounting the roller above the support plate. The two cycloids (inverted for clarity) represent a point on the underside of the roller and therefore the pendulum bob With the rod above the plate we get "A" With it below, we get "B"

dave8

p.s. can our helpful moderator turn the image olease?

Edited By SillyOldDuffer on 28/08/2023 21:45:31
30 August 2023 at 14:04 #658493
david bennett 8
Participant
@davidbennett8
A first quick and dirty try. The rod is 840mm by 3mm. The magnets and coins are as shown earlier. There is a good rolling action, but the rod is much to thin and long, allowing too much error in the bob (figure of eight) The amplitude falls from 90mm to 45mm in about two minutes. Such a terrible Q is not surprising, considering the work the pendulum has to do, and the rolling surfaces are so far unmachined. The magnets are very strong, and would hold a much heavier bob.

dave8

,

Edited By david bennett 8 on 30/08/2023 14:06:37
30 August 2023 at 18:27 #658533
SillyOldDuffer
Moderator
@sillyoldduffer
A gripping result!

I'm having trouble conceiving how it works. Presumably low Q is caused by friction between coins and top-plate resulting from the magnetic force. I wonder if Q could be improved by increasing bob weight until gravitational force almost balances the magnetic force.

I'm guessing friction follows the same rules whatever type of force brings two moving objects into contact. Does anyone know?

Dave
30 August 2023 at 20:51 #658558
david bennett 8
Participant
@davidbennett8
Dave, don't let the coins fool you. They are the modern 2p coins that contaiin iron. As it is a rolling action, it doesn't feel frictiony. It should improve when the coins are replaced by chamfered discs to mimic knife-edges. Bear in mind the rolling action means the effort to physically lift the bob must come from the pendulum. I have ordered some differen sized magnets to hopefully tune the attraction. Also, a half second-sized rod might be better.

dave8
30 August 2023 at 22:39 #658572
Tony Jeffree
Participant
@tonyjeffree56510
Intriguing idea.

Just a thought – but would it help in tuning the behaviour of this setup if the coins/discs were running on a curved (rather than flat) track?
30 August 2023 at 23:30 #658576
david bennett 8
Participant
@davidbennett8
Posted by Tony Jeffree on 30/08/2023 22:39:50:

Intriguing idea.

Just a thought – but would it help in tuning the behaviour of this setup if the coins/discs were running on a curved (rather than flat) track?

Afraid not. The whole concept is to mimic a cycloidal path, which is defined by a circle rolling on a straight (flat) plane.

dave8

Edited By david bennett 8 on 30/08/2023 23:31:33
31 August 2023 at 02:58 #658581
david bennett 8
Participant
@davidbennett8
A small improvement – by using 1p coins and only 2 magnets half amplitude is about 3 minutes. At least it suggests a way forwards.

dave8
31 August 2023 at 07:25 #658590
John Haine
Participant
@johnhaine32865
To be clear, there is only one cycloidal path that makes the pendulum isochronous and that's one where the roller radius (or diameter?) equals the pendulum length. Anything else just approximates the cycloid for small angles in a way that can correct the isochronism. Anyway the path of the bob for this setup is epi-trochoidal. As far as I know everyone who has looked at this (mainly Woodward) just assumed that the magnetic version would be impracticable and the calculations of the required roller diameter haven't been done, you're pioneering here Dave! Also one would have to factor in that real pendulums are compound just to make it more interesting.
31 August 2023 at 07:47 #658591
Michael Gilligan
Participant
@michaelgilligan61133
Posted by John Haine on 31/08/2023 07:25:50:
.

To be clear, there is only one cycloidal path that makes the pendulum isochronous and that's one where the roller radius (or diameter?) equals the pendulum length. […]

.

As described by Huygens in the page that I posted 28/08/2023 16:15:31

”Upon a flat table … “

MichaelG.
Author

Posts