I seem to remember that RF runs on the surface of conductors so you do not need solid rod copper. I made lots of tuning devices for a certain department who monitored the ether, silver was the preferred choice of materiel and surface. It will give you some gain.

I am sure there are some plating shops that will do it. check the internet for one.

Clive

Hi Steve,

This 2012 paper describes a cyanide free mix and how to use it.

Also these chaps sell a cheaper commercial mix than the source you found. Their site also has a calculator for working out how much liquid you need and lots of other help re cleaning and voltages etc.

Dave.

Can't help with the silver plating but I can give some pointers on high frequency performance:

The resistivity of copper is 1.72 microohm/cm, giving a skin depth at 1.3 GHz of 1.83 micron.

The resistivity of silver is 1.59 microohm/cm, giving a skin depth at 1.3 GHz of 1.76 micron.

So, while the resistivity of silver is lower the current flows in a thinner layer and the improvement is less than you might expect. In fact the resistance will only drop by 4% and the Q factor of the resonators will thus only improve by 4%.

Silver plating can give a long term benefit as the tarnish on copper can be semiconducting however a thin coating of lacquer is easier and cheaper to apply.

Russell

Edited By Russell Eberhardt on 25/03/2017 20:31:37

Edited By Russell Eberhardt on 25/03/2017 20:34:00

Further to the excellent quidance from Russell:

For loss in synchronously tuned filters, in the time when I was (less) gainfully employed, a couple of extremely useful reference works were written by Seymour Cohn, specifically see ‘Dissipation Loss in Multiple-Coupled-Resonator Filters’, Proc. IRE August 1959 if you can find access.

I don’t now have access to my paper copy, but I remember something close to:

Filter loss in dB = 4.343*(reciprocal of fractional BW)*sigma(gi/Qi)

Where Qi is the Q factor of the i’th resonator, and gi is the normalised value of the i’th resonator (for the low pass prototype at a frequency of 1 radian/sec and impedance 1 ohm). I cannot remember whether the fractional BW relates to the half-sided BW or the full BW as a ratio of centre frequency.

But anyway, the passband insertion loss in dB is directly proportional to the inverse of the resonator Q factor (simplifying to assume the resonators are all the same). The stopband performace is not significantly affected by the Q factor.

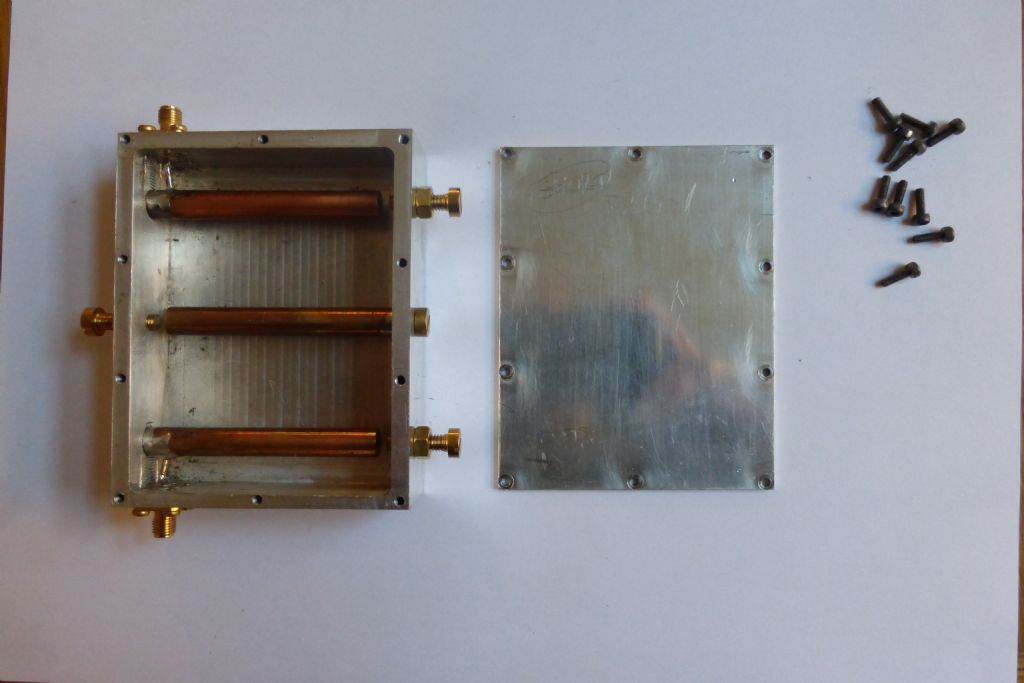
Note that silver oxide is electrically conductive (hence silver use in switch contacts and general electronic use), but copper oxide is significantly less so, see use in copper oxide rectifiers, **LINK**

I agree that coat of lacquer will give much of the long-term benefit of silver plating

Martin

Edited By Martin Whittle on 26/03/2017 20:53:29

Has it actually been established that any silver plating solution uses cyanide? I used to be involved with plating tubular spacecraft antennas in the 70's using both gold and silver. I distinctly remember cyanide being used in the gold-plating process but I don't recall it in the silver process.

….. but it was along time ago and I was only peripherally involved in the actual plating of the antennas.

Steve, from Henley's Formulas For Home and Workshop; 1907, rev.1927 reprint 1979,with suitable warnings on the frontispiece. (ISBN- 0-517-293072)

1) Nitrate of silver-30 parts by weight, Caustic potash-30 parts, distilled water 100 parts. Pour the nitrate into the water, one quarter hour after, add the potash. When the solution is done, filter, it is sufficient to dip the parts into the bath, moving them for 1-2 minutes at most; then rinsing and drying in sawdust. It is necessary to pickle the pieces before using the bath. To make the nitrate of silver ones self, take 30 parts of pure silver and 60 parts nitric acid, and when the metal is dissolved, add the caustic potash and the water,

2) Pastes for silvering; carbonate of lime-65 parts, sea salt-60 parts, cream of tartar-35 parts,nitrate of silver-20 parts. Bray all in a mortar, not adding the carbonate of lime until all other substances are reduced to a fine powder. Next add a little water to form a homogeneous paste, which is preserved in blue bottles away from the light. for use, put a little of this paste on a small pad and rub the article with it.

Most of their other methods use cyanide.

The book does make good bedtime reading though.

I do like the way this forum challenges my perceptions. I've been a radio amateur since I was 16 and thought I knew all about silver plating RF components and why it's done. Now a bit of research reveals that all the alleged benefits are questionable. Arghh!

The strange thing is that expensive well made RF gear 'always' contains silver, sometimes lavishly. It's not for show because much of it is shielded, hidden away in the works. The user doesn't know it's there.

So why is it done? I'm starting to suspect that even engineers, who should know that "quality" is meaningless without a well specified purpose, may have fallen into "the expensive one must be the best" trap. Or is it just that the 'obvious' benefits of silver's low conductivity became 'industry best practice' and no-one's ever challenged it?

But surely my paranoid logic can't apply to mobile phones. They're full of precious metals that their buyers don't know or care about…

I'm thoroughly confused!

Dave

By the way, there's general agreement that powder applied silver doesn't help at RF. The silver has to be in a solid layer. The gaps between particles of powdered silver aren't conductive.

.

I think that it's because electro-plated Silver is of consistently high purity and quality … whereas the undelying Copper is almost certainly not super-dooper mono-crystalline stuff.

MichaelG.

Ahem, skin depth may not change, but a diameter increase of 1% means circumferential change of 3.14%m, therefore an area change of 3.14%

Neil

Circumference = pi x D

pi is constant so circumference is proportional to D

Russell