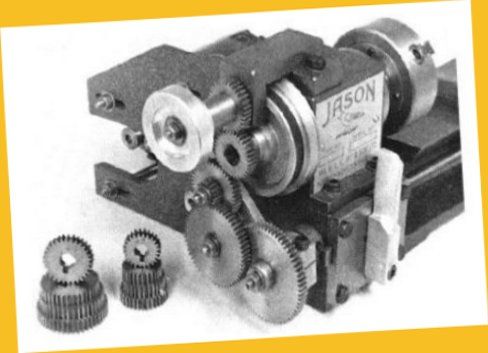
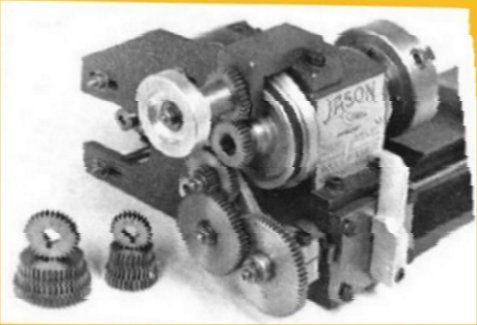
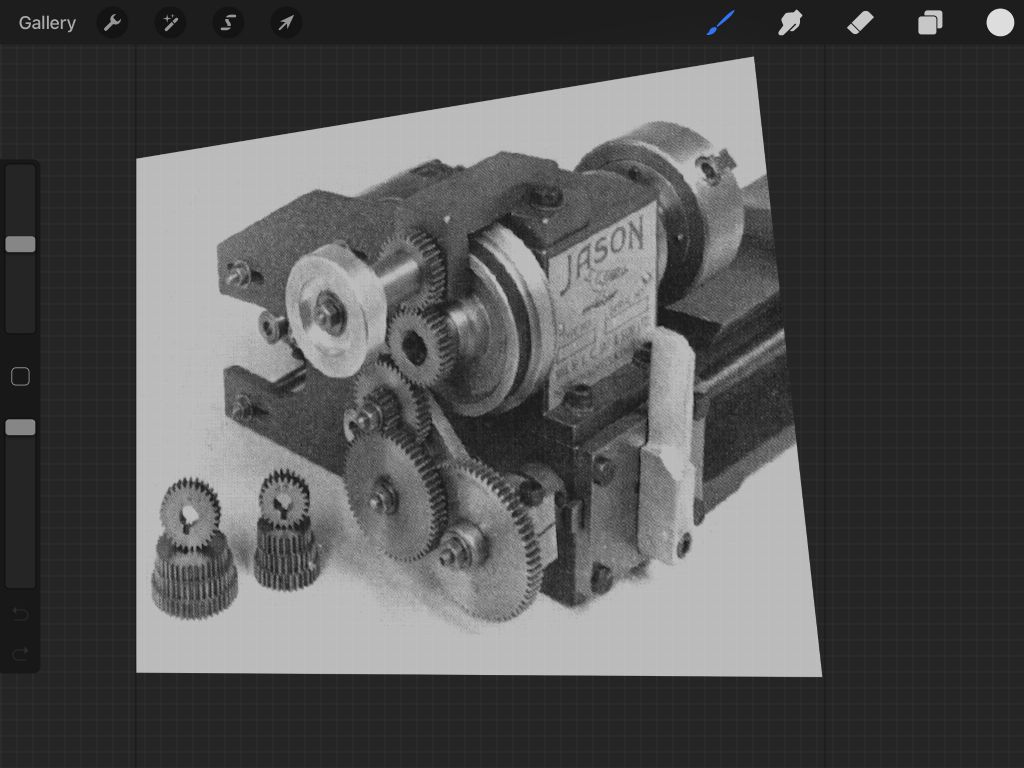
In search of the ‘reverse algorithm’
In search of the ‘reverse algorithm’
- This topic has 21 replies, 7 voices, and was last updated 3 June 2021 at 23:54 by
 Michael Gilligan.
Michael Gilligan.
Viewing 22 posts - 1 through 22 (of 22 total)
Viewing 22 posts - 1 through 22 (of 22 total)
- Please log in to reply to this topic. Registering is free and easy using the links on the menu at the top of this page.
Latest Replies
Viewing 25 topics - 1 through 25 (of 25 total)
-
- Topic
- Voices
- Last Post
Viewing 25 topics - 1 through 25 (of 25 total)
Latest Issue
Newsletter Sign-up
Latest Replies
- Measuring a double Vee lathe bed Vee position
- Please direct me to where I can find an engineer to do some bespoke work
- Making Unimat DB/SL Steadies
- Bad design, or am I missing something?
- Lidl castors
- Hopeless…Alibre Ass
- Polishing compounds for stainless steel (mild abrasives))
- Alternative to ARC
- My vise isn’t at 90 degrees
- Sat nag